Calculadora en línea: Volumen de un tanque cilíndrico inclinado

Para realizar el cálculo debes ingresar el tamaño del tanque, ángulo y nivel de líquido.

Medición del nivel del líquido

Debes medir el nivel de líquido en la línea media del tanque perpendicular al fondo del tanque. (ver la imagen). Puedes medir el nivel del líquido a cualquier distancia de la base (Si lo haces, debes ingresar la distancia en un parámetro especial).
De forma alternativa, puedes inclinar el tanque para crear un nivel cero de líquido en la base superior, en este caso debes sólo medir el ángulo de inclinación.

Debajo de la calculadora se encuentran detalles del cálculo y fórmulas.

Volumen del líquido en un tanque cilíndrico inclinado

Longitud del cilindro

Radio del cilindro

Ángulo de inclinación (grados)

Nivel de líquido

Nivel medido cerca de la

base superior

base inferior

Distancia de la base

Distancia entre el nivel de líquido medido y base cercana. (0 si se midió cerca de la base)

Cálculo preciso

Dígitos después del punto decimal: 2

Nivel del líquido cerca de la base superior

Nivel del líquido cerca de la base inferior

Longitud de la parte del tanque parcialmente llena

Longitud de la parte del tanque completamente llena

Volumen del líquido

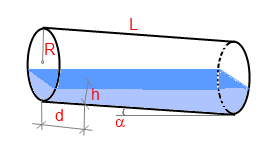
No puedo encontrar una solución ya hecha para calcular el volumen de un líquido en un cilindro inclinado, así que derive la fórmula de esta forma:

$V = \frac{R^2}{2}\int_{\small0}^L {\theta(x)-\sin{\theta(x)}dx$
donde $\theta(x)$ - como el ángulo del segmento depende de la longitud x del cilindro,
se puede derivar como:
$\theta(x)=2\arccos{\frac{R-h}{R}}=2\arccos{\frac{R-(x\tan{\alpha}+h_{0})}{R}}$
donde
a - ángulo de inclinación,
h0 - nivel de líquido en la parte superior

Si substituimos esta expresión en la fórmula, obtenemos:
$V = R^2\int_{\small0}^L {\arccos{u}-u\sqrt{1-u^2}dx=-\frac{R^3}{\tan{\alpha}}\int_{\small{u_0}}^{u_L} {\arccos{u}-u\sqrt{1-u^2}du$
donde $u=\frac{R-(x\tan{\alpha}+h_{0})}{R}$

Si tomamos la integral obtenemos la solución:
$V = -\frac{R^3}{\tan{\alpha}}\left( u\arccos{u}-\frac{1}{3}\sqrt{1-u^2}(u^2+2)\right)\large|_{\small{u_0}}^{u_L}$
$= \frac{R^3}{\tan{\alpha}}\left( K\arccos{K}-\frac{1}{3}\sqrt{1-K^2}(K^2+2)-\left[K-\frac{{L}tan{\alpha}}{R}\right]\arccos{\left[K-\frac{{L}tan{\alpha}}{R}\right]}+\frac{1}{3}\sqrt{1-\left[K-\frac{{L}tan{\alpha}}{R}\right]^2}\left(\left[K-\frac{{L}tan{\alpha}}{R}\right]^2+2\right) \right)$